Momentum and Gravity

Gravity - we all know, but the other behaves very differently. With momentum, you can overcome gravity itself.
Gravity is the most well-known phenomenon on this earth. Everything is subject to it. Whether it be walking up and down hills or falling down the stairs or even kneeling down and standing back up again, gravity pulls us to the ground. Growing up with it from birth, and designed to need such gravity to do the basic functions we take for granted, we understand it even if we don’t know how it truly works.
Gravity works by two objects being in proximity together. The larger their mass, the greater the gravitational force. Small objects, like two pens, have negligible gravitational pull to each other, even in the emptiness of space, with no outside forces applied to them, they would barely affect each other. However, large objects, such as a planet and its inhabitants, have a significant effect on each other.
Newton’s formula for the universal law of gravity is F_G=(Gm_1 m_2)/r^2, where fg is the force of gravity, G is the universal gravitational constant (6.6726 x 10-11N-m2/kg2), m1 and m2 are the two masses of the objects (in kilograms), and r2 is the square of the distance between each object’s radius (in meters). For instance, an 80kg (176.4lb) man standing on the surface of earth at the equator, at approximately sea level, is 6,378km from the center of the earth (NASA's Goddard Space Flight Center). Earth is a very dense planet, with an estimated weight of 5.9722 x 1024 kg.
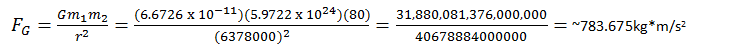
Momentum is also very well-known, and even if you don’t know it technically, you most certainly have experienced it. In a car that is braking hard, you feel yourself pressed forward. When turning, you can feel yourself being pushed away from the ‘inside’ of the corner. This is sometimes mistakenly called inertia.
Inertia is a characteristic all objects have, the characteristic of resisting change in motion, based solely on the object’s mass. Momentum is a force when an object is moving in a direction, based on mass AND velocity. Because of this, it is your own momentum that is pushing you around.

However, the kind of momentum needed to stimulate gravity is known as angular momentum. Being pressed in a straight line requires us to accelerate at a constant rate to feel something akin to gravity. However, with angular momentum, we can feel a force like that of gravity due to a constantly changing direction, rotated around a point with a constant radius. Angular momentum is simply the amount an object rotates along with their speed.
A personal favorite ride, and a perfect example, is the “Gravitron” (aka “Starship #000,” “Twister,” “Area 51,” etc.). A simple UFO spins its riders against the wall at 3 times the strength of gravity, allowing people to lay, sit, or even stand against the wall, completely perpendicular to gravity. It is literally a mild human centrifuge.
The walls are angled upwards, rather than completely flat, to allow its riders to lift up from the ground, and feel as though gravity has been completely cancelled. Had the 3G’s of force been applied to a flat surface (such as the “Round Up” ride pictured below), the rider would still feel the effect of normal earth gravity. Due to the mild angle, riders are pressed upwards a small bit more by momentum than they are pulled downwards by gravity, resulting in the lift. Let’s see if we can calculate their angular momentum, ignoring the slight angle.

Let’s calculate the angular momentum of an 80kg man on the Gravitron, pressed against the wall 3 meters away from the axis of rotation, and is at the ride’s maximum speed of 24rpm.

L = Iω, where L is angular momentum, I is the moment of inertia (kg∙m2), and ω is the angular velocity (radians ÷ s). The moment of inertia for this situation is defined as m*r2 (mass*radius2) and ω is v/r (velocity ÷ radius) which simplifies to mrv (mass*radius*velocity). Before we begin, we must convert RPM to radians per second, which is rpm *2π/60 , (which simplifies to π/30 ). This means our speed is 24π/30 or 0.8π r/s.
L = mrv = (80)(3)(0.8π) = 192π = 603kg*m/s
Huh, it looks like gravity is still stronger than the ride itself! Well, if you were sharp, you noticed a difference in the units we ended up with: kg*m/s2 is not the same as kg*m/s! That’s because gravitational acceleration and angular momentum are not the same thing. Sure, the ride makes you feel you’re being sucked back against the wall harder than gravity ever pulled you down, but it’s with speed, not acceleration! We can roughly equate this angular momentum to three times gravity’s pull, but it’s not a direct comparison.
About the author: Robert Wich is pursuing Bachelor of Mechanical Engineering degree at FIU. He is an avid car enthusiast. In his spare time, he dismantles automobiles and puts them back together. He is also interested in car design and has a plenty of sketches to support.

He teaches Math and English. With his help, many of his students achieved proficiency Math in a short time and graduated out of high school.

About Us
FPLA is Miami’s premier tutoring center in reading, math, science, and test preparation programs. At FPLA, we encourage your child to aspire to a bright future by empowering them with academic and life skills in a positive learning environment that is equipped with a variety of learning resources. Now, you can get the same one-on-one tutoring in live online classes as well.
